Patterns in Sight Reading Preferences

It’s possible to gain an incredible fluency at music reading, absorbing large sections of music at a glance. I remember as a youngster being in awe at those who could instantly transform squiggles into beguiling sounds, as if there was no barrier between the symbols, the musical brain and the appropriate finger movements. Even without an instrument to hand, they could somehow hear the page. However, music reading seems to differ from word reading in that it can be quite easily tripped up. With word reading you can read wrds wtht vwls frly cnfdntly, or when they are uʍop ǝpᴉsdn, or even ndsᴉpǝ poʍu ɐup qɐɔʞʍɐɹps. However the odd enharmonic or dotted rather than tied note in music notation, can momentarily derail even an experienced reader.

What I’ve found puzzling is that there is no one unanimous convention for notation, nor a system that will please all the people all the time. So in this short article I will attempt to reveal some of music notation’s hidden conventions and reader preferences.

Some disclaimers:

• This is a ‘study’ of a mere 54 participants, ranging from intermediate to highly experienced note readers. All from Facebook, and most of whom are my friends, so make of that what you will.
• We are limiting ourselves to 4/4, single notes and no rests. A major restriction, but one must start somewhere.
• This is very lazy research. I spent a few seconds googling relevant terms, but basically wanted to follow through these ideas prima vista. I’m sure if I looked for 7 seconds longer I’d find Ideathief & Twatamaholey’s comprehensive and beautifully argued 1973 thesis on the topic. So be it.
• I have no affiliation with Big Quaver.

Ok so Figure 1, shows a series of numbered extracts, from which the subject indicates a preference (or no preference).

Figure 1: The Test

You may want to make a note of your preferences at this point, before we look at the results from our cohort of readers.

We’ll break these down in sections so we can discuss the various implications.

The Extent of Imaginary Barlines

Examples 1-3 test the concept of the imaginary barline – the idea that we should notate as if 4/4 was actually 2 consecutive 2/4 bars, separated by an invisible barline. Of course, semibreves (and dotted minims) violate this regularly, so we might consider them honorary exceptions to this rule. But this begs the question, at what point does the imaginary barline ‘kick in’?

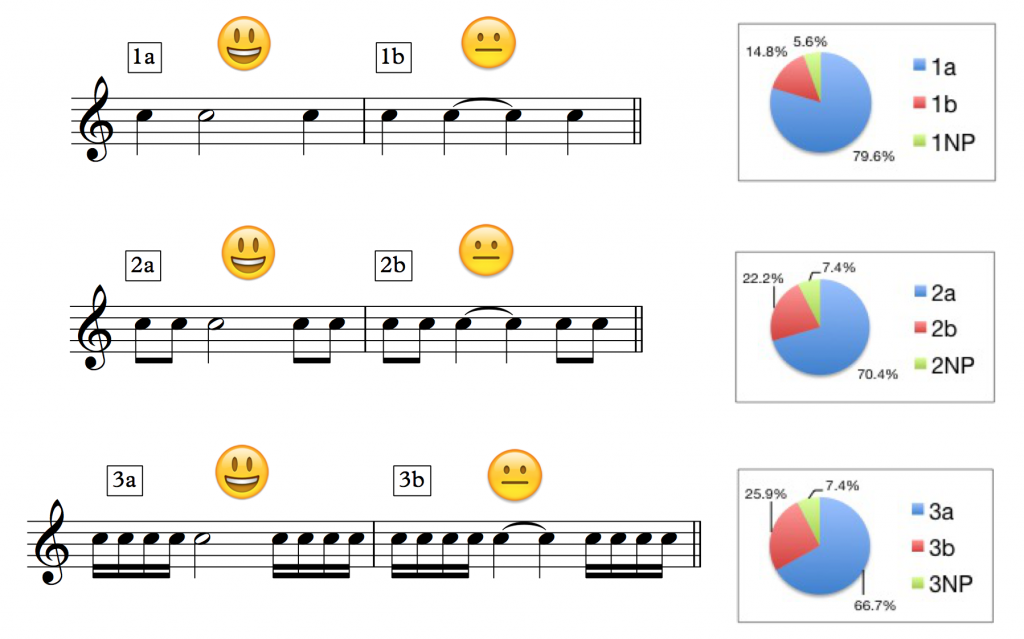
Figure 2 presents the results of the first 3 exercises

Figure 2 Preferences for Examples 1-3, testing the limits of the imaginary barline. Notice how preference is eroded by the weaker ‘bridge posts’. (NB NP= no preference and please note also that due to rounding errors, the percentages in this – and other examples – may not add up to 100% exactly, please get over it.)

You’ll notice that in all 3 cases, a central minim is preferred over an imaginary barline. At this point I’m going to suggest some terminology, let’s call the formation with a central minim (covering beats 2 and 3) as a minim bridge. It seems that readers tolerate this well. Note that positing the minim bridge allows us to talk of – among other things – crotchet bridges on beats 1& to 2& and their implications in sight reading.

Notice that the preference for the minim bridge reduces as we subdivide the material on beats 1 and 4). 1a has a minim bridge well supported by a crotchet post on either end, clearly presenting beats 1 and 4. 1b and 1c on the other hand have weaker posts, the quavers and semiquavers respectively, slightly masking the clarity of beats 1 and 4.

We could in fact imagine minim bridges of different strengths. While the majority may prefer a minim bridge to an imaginary barline (IB), as the material at beats 1 and 4 become more complex, perhaps more people prefer the IB over the bridge presentation (see Figure 3)

Figure 3 Hard and weak minim bridges with imaginary barline equivalents, and a suggested preference curve. (Click to embiggen)

The limited data supports this, but it’s only a tentative suggestion. Furthermore, I suspect that there will be a significant proportion of readers who will always prefer (or at least tolerate) a minim bridge regardless of the content in the rest of the bar.

Bridge Stability

So far we’ve seen that there is a general preference for minim bridges over imaginary barlines, less so perhaps as the minim’s supporting posts on beats 1 and 4 become more complex. But what of the minim bridge itself? How robust is it to any complexities? The answer, it seems, is not very. Figure 5 shows that when the minim bridge is divided into a quaver-crotchet-quaver pattern (a crotchet bridge formation), preference swings dramatically to the imaginary barline presentation, and slightly more so when the beats 1 and 4 posts are made more complex. So a fractal//Inception-style bridge-within-a-bridge is too much for many readers to bear, although a good 1 in 4 readers actually preferred it over IB. I suspect that some readers, don’t need any IB at all, and are happy to see a sequence of rhythmic values with no visual indication of beat 3. Let’s call these readers who can tolerate (or prefer) a sequence of rhythmic values over IB presentations as ‘sequential readers’.

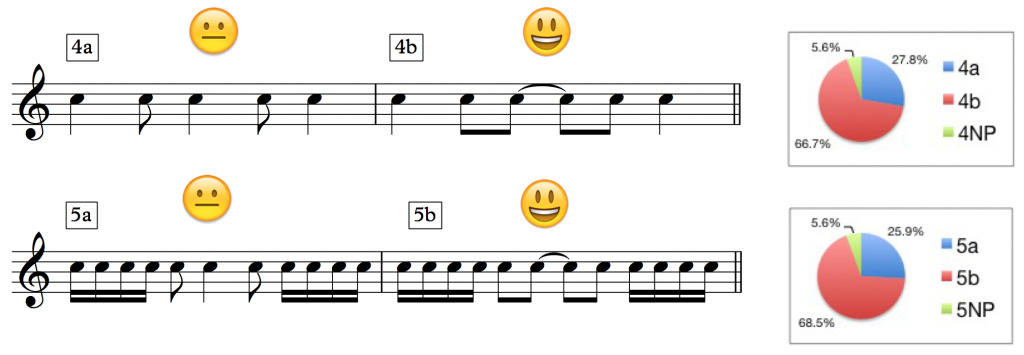
Figure 4. A minim bridge collapses for most people when it is subdivided into a crotchet bridge. Still, those 1 in 4 sequential readers prefer it to IB.

 Crotchet Bridges and Imaginary Quarter-Barlines

So we’ve dealt to some degree with minim bridges and their relationship to the imaginary barline, and their kryptonite (the fractal bridge), but what of bridges on beats 1 and 2, and beats 3 and 4? In other words, do the same principles scale down from the whole bar to the half bar?

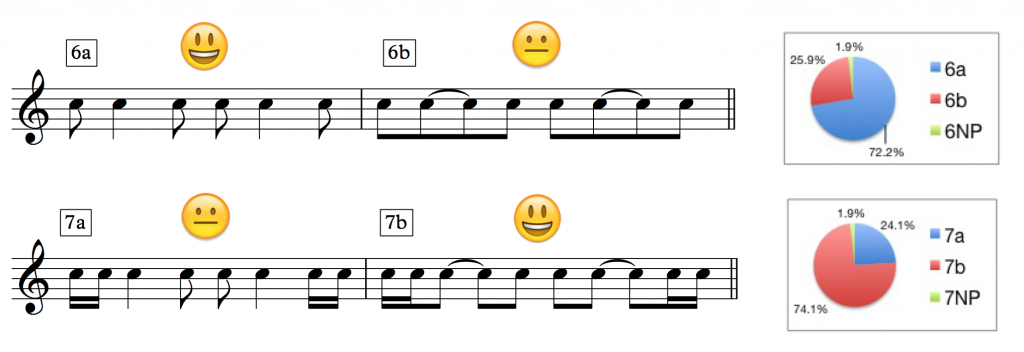
Figure 5 Testing the Crotchet Bridge (or crotchet IB)

Examples 6 and 7 address this question. You’ll see in Figure 6 that the majority prefer the crotchet bridge to (beat 2 and 4) imaginary barlines (6a over 6b) although this preference is less common than at the whole bar level (1a over 1b). However the crotchet bridge (surprisingly to me) seems far more susceptible to weakening than the minim bridge. Notice that as soon as we weaken the posts on the first and last quavers, preference sways over dramatically to the crotchet IB (7b over 7a). This was just a tentative study (quiz really) but if I knew of this phenomenon I would have interrogated the extent and nature of the fragile crotchet bridge more thoroughly.

Quaver Bridges, Patronizing Over-information and Even More Imaginary Barlines

Let’s look now at the quaver bridge (semiquaver-quaver-semiquaver). Figure 6 shows that a similar ratio of readers prefer quaver bridges to minim bridges (8a over 8b, as compared to 1a over 1b). However some subjects reported (without solicitation) that 8b was ‘horrible’ or ‘horrifying’. Why this ‘over-information’ created such a negative reaction (as compared to 1b) is not clear. It could be that it is simply the case of 4 instances over 1, or the shortened time scale is less tolerant of unnecessary fuss. I suspect it’s the sequential readers kicking up the fuss, I’ll check.

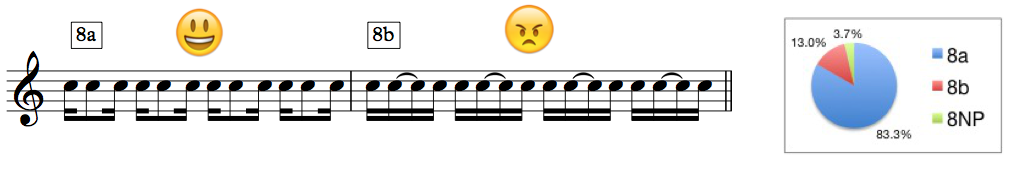
Figure 6 How to piss off a sequential reader.

The quaver bridge also allows us to check if the standard IB (splitting 4/4 into 2 2/4 bars) is sufficient once semiquavers are introduced. You’ll see in Figure 8 that in fact a slight majority of readers prefer to have imaginary barlines on every beat in the presence of semiquaver syncopation. A good portion preferred standard IB but one who didn’t was upset by the crotchet IB violation. He’ll recover in time.

Figure 7 Half vs. quarter imaginary barlines with semiquaver syncopation

Let’s now test sequential vs. standard IB vs crotchet IB in a syncopated semiquaver passage (Figure 9). The majority preferred crotchet IB (essentially 4 imaginary bars of 1/4), but a significant portion were happier with either no IB or standard IB presentations (Incidentally the former upset some readers presumably because it was the opposite of patronizing). Why did more prefer 10a to 10b (given 10c’s majority) is a bit puzzling. Perhaps there is an in-for-a-penny-in-for-a-pound sentiment in operation here (“if I don’t need one tie over beats 2 and 3, I don’t need any other”).

Figure 8 No vs. standard vs. crotchet IB with semiquaver syncopations.

Additive Meters vs. Imaginary Barlines

Aside from minim bridge and the trivial semibreve and dotted minim cases, there are relatively common violations of standard IB, in the context of additive meter. To test this, let’s compare preferences for 3+3+2 vs. 2+3+3 vs. 3+2+3 quaver groups. All of these violate standard IB but will any be preferred over it. In every case standard IB is preferred (11b, 12b and 13b), but around 20-30% went for the ‘full additive’ version. This was particularly true in the 3+3+2 formation rather than the other permutations (one of which was ‘offensive’ to some). Why would this be the case? They all violate standard IB so what else differentiates them? It may be that 3+3+2 is the most commonly seen of these meters (Butler 2006) or – and perhaps because – it has a clear beat 4 ‘post’ to anchor the reader. You’ll notice that readers clearly preferred standard IB over crotchet IB (11b, 12b and 13b over 11c, 12c and 13c).

It perhaps hits the sweet spot between rhythmic clarity and patronizing over information.

Figure 9 Additive meter vs. imaginary barlines

Conclusions

This was a limited, uncontrolled study glorified quiz with a small number of participants, all of whom could see each other’s responses. Still, useful (and surprising) concepts emerged. In particular, I think there is mileage in terms of the bridge concept, and a more flexible concept of the imaginary barline which adapts dynamically to subdivision and syncopation, as well as the sweet spot between fussy information and pure sequentualism. I’m also going to investigate preference patterns and consistencies (or otherwise) within (rather that between) subjects’ responses.

The preferences here may be merely suggestive of experience and convention (although how these conventions emerge is interesting of itself). It’s unlikely that our preferences are the results of evolutionary pressure – quick-read-this-syncopated-passage-to-distract-the-tiger scenarios – but it may tell us something about our music reading faculties, and whether an inexperienced notator’s (and Logic notation) can be so baffling . Whether these preferences are internally consistent or optimal may be questioned, but it’s useful to actually know what a majority of readers prefer when preparing scores. So let’s ask them (ourselves).

Many thanks to those who gave up their time and composure in completing this test. Very interested to hear your preferences, and any feedback below.