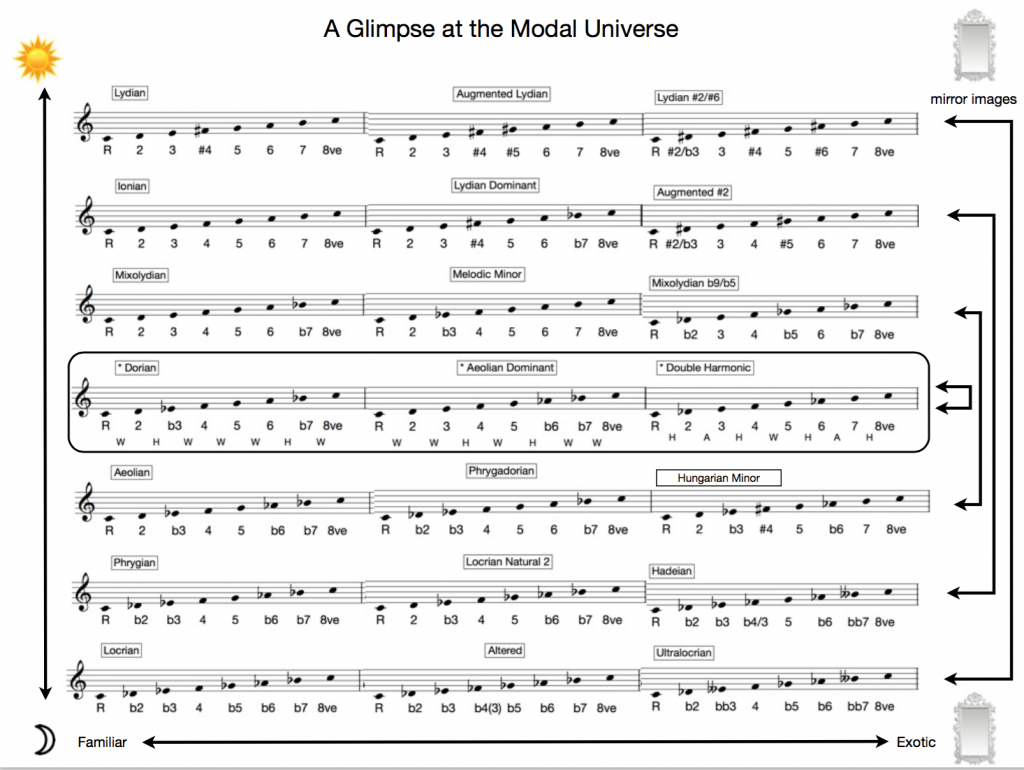
A diagram demonstrating a section of the huge modal universe. You may see how mirroring modes (turning them upside down) can organize them into levels of brightness. It can also identify those modes that are identical in mirror form. These include Dorian (used in a thousand tunes from Scarborough Fair, Shine on You Crazy Diamond, Brick House to The Hitchhiker’s Guide To The Galaxy), Aeolian Dominant (Babooshka) and Double Harmonic (Miserlou from Pulp Fiction).
These are just 3 of the heptatonic even-tempered modes with mirror symmetry parents. There are many others, scales with 2-12 notes, as well as scales with ‘twin’ mode systems. Regardless this technique can be applied widely and is a rich resource for composers and improvisers alike.
(Visited 194 times, 1 visits today)

8 replies on “A Glimpse At the Modal Universe”
Hi!
What do you mean by “twin” mode systems?
Thanks
Hey G,
Ok so is we invert and mode of the major scale system, we get a mode of the major scale system (Locrian makes Lydian, Phrygian makes Ionian, Aeolian makes Mixolydian (and the reverse of course). Dorian remains unchanged). It’s a closed system. Same is true of the modes of melodic minor and the Miserlou. However if you invert and mode of the Harmonic minor (for example), it does not become a mode of the harmonic minor scale. It’s not a closed system. They in fact become modes of the Harmonic major scale (and of course the reverse is true). This is what I mean by “twin mode systems”. The harmonic minor and major modal groups are twinned through inversion. I hope that helps you in some way.
cool! awesome thanks!
Ya that’s really cool. This reminds me of what people are referring to these days as negative harmony, which is a really cool concept. Hmmm… I wonder if there is a logic in what types of structure/scales will stay closed or “twin”. (I guess those minor third jumps have something to do with it. )
that’s really cool, I’m going to mess around with those ideas, thanks!
You’re welcome!
I think what determines closed/openness (and I’ve only just thought of this prompted by your good question) is the existence of a palindromic mode within the group. Dorian and Hindustani are both palindromic and belong to (separate) modal groups. Same goes the Miserlou despite having (crucially 2) augmented 2nd gaps. The harmomic minor (and harmonic major) has only one augmented second gap so can’t have a palindrome in the group so can’t be closed. If you think about the 3 semitones you can’t put a mirror on them to make it look identical (unless you use a quarter tone!) so another 3 semitones gap is needed for the possibility of palindromic-ness. Thanks for the question!
ah right, 1 palindromic mode.
And to think of it, I guess a palindromic mode would be inversely symmetrical above and below the tritone….
ie dorian is symmetrical moving stepwise both upward and downward starting from the tritone
(mixo #11 as well is symmetricall from the tritone)
i think that holds true, just thinking off the top of my head.
cool stuff!!
sorry, mixo b13
Could not follow what “mirroring modes” entails (except perhaps between Lydian and Locrain – which have different accidentals/naturals for every note – except for their tonics). However, the arrangement of modes from bright to dark and from familiar to exotic provides a useful birds-eye view of a wide range of modes.
By ‘mirroring’ imagine a scale starting on C rising above the surface of water with C At sealevel’. The reflection reverses the order of intervals: so C to D (tone up)creates C down to Bb (tone down) in mirror scale. Doing so turns Lydian to Locrian (and vice versus) Ionian TO Phrygian, mixolydian to aeolian.
Dorian is the same in mirror form because its intervals (of whole and half steps (WH))are a palindrome WHWWHWWHW
Quite beautiful